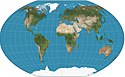
Liste von Kartennetzentwürfen

Dies ist eine chronologisch sortierte Liste von Kartennetzentwürfen, die eigene Artikel auf Wikipedia haben oder anderweitig bemerkenswert sind. Da die Anzahl der möglichen Kartenprojektionen nicht begrenzt ist,^[1] kann es keine umfassende Liste geben.

Erläuterungen

Projektionstyp

Zylindrisch

In der Standarddarstellung bilden diese regelmäßig beabstandete Meridiane zu gleichmäßig beabstandeten vertikalen Linien und Parallelen zu horizontalen Linien ab.

Pseudozylindrisch

In der Standarddarstellung bilden diese den Zentralmeridian und die Parallelen als gerade Linien ab. Andere Meridiane sind Kurven (oder möglicherweise gerade vom Pol zum Äquator), die regelmäßig entlang von Parallelen verlaufen.

Kegelförmig

In der Standarddarstellung bilden konische (oder kegelförmige) Projektionen Meridiane als Geraden und Parallelen als Kreisbögen ab.

Konisch

In der üblichen Darstellung stellen konische Projektionen die Meridiane als Geraden und die Parallelen als Kreisbögen dar.

Pseudokonisch

In der Standarddarstellung stellen pseudokonische Projektionen den Zentralmeridian als gerade Linie, andere Meridiane als komplexe Kurven und Parallelen als Kreisbögen dar.

Azimutal

In der Standarddarstellung stellen azimutale Projektionen Meridiane als Geraden und Parallelen als vollständige, konzentrische Kreise dar. Sie sind radial symmetrisch. In jeder Darstellung (oder in jedem Aspekt) bewahren sie Richtungen vom Mittelpunkt aus. Das bedeutet, dass Großkreise durch den Mittelpunkt durch Geraden auf der Karte dargestellt werden.

Pseudoazimutal

In der Standarddarstellung bilden pseudoazimutale Projektionen den Äquator und den Zentralmeridian auf senkrechte, sich schneidende Geraden ab. Sie bilden Parallelen zu komplexen Kurven ab, die vom Äquator weg verlaufen, und Meridiane zu komplexen Kurven, die zum Zentralmeridian hin verlaufen. Hier nach pseudozylindrisch aufgelistet, da sie ihnen in Form und Zweck im Allgemeinen ähnlich sind.

Retroazimutal

Die Richtung zu einem festen Punkt B (auf dem kürzesten Weg) entspricht der Richtung auf der Karte von A nach B.

Andere

Typischerweise aus einer Formel berechnet und nicht auf einer bestimmten Projektion basierend.

Polyhedral

Polyedrische Karten können zu einer polyedrischen Annäherung an die Kugel gefaltet werden, wobei eine besondere Projektion verwendet wird, um jede Fläche mit geringer Verzerrung abzubilden.

Eigenschaften

Konform

Bewahrt die Winkel lokal, was bedeutet, dass lokale Formen nicht verzerrt werden und dass der lokale Maßstab in allen Richtungen von jedem gewählten Punkt aus konstant ist.

Flächentreu

Das Flächenmaß bleibt überall erhalten.

Kompromiss

Weder konform noch flächentreu, sondern ein Gleichgewicht, das die Gesamtverzerrung reduzieren soll.

Äquidistant

Alle Abstände von einem (oder zwei) Punkten sind korrekt. Andere äquidistante Eigenschaften werden in den Anmerkungen erwähnt.

Gnomonisch

Alle Großkreise sind Geraden.

Tabelle chronologisch

Chronologie der kartographischen Projektionen

Abbildung	Jahr	Projektion	Urheber	Typ	Eigenschaften	Anmerkungen
	580 v. Chr. (ca.)	Gnomonische Projektion	Thales (möglicherweise)	Azimutal	Gnomonisch	Alle Großkreise werden auf Geraden abgebildet. Extreme Verzerrung weit vom Zentrum entfernt. Zeigt weniger als eine Hemisphäre.
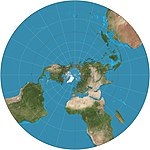	200 v. Chr. (ca.)	Stereografische Projektion	Hipparchos * (veröffentlicht)	Azimutal	Konform	Die Karte ist unendlich groß und die äußere Hemisphäre bläht sich stark auf, so dass sie oft als zwei Hemisphären verwendet wird. Kartografiert alle kleinen Kreise zu Kreisen, was für die planetarische Kartierung nützlich ist, um die Formen von Kratern zu erhalten.
	200 v. Chr. (ca.)	Orthografische Azimutalprojektion	Hipparchos * (veröffentlicht)	Azimutal		Blick aus unendlicher Entfernung.
	100 (ca.)	Längentreue Kegelprojektion = (äquidistante od. auch isometrische) = einfach kegelförmig	Basierend auf der ersten Projektion von Claudius Ptolemäus	Konisch	Flächentreu	Die Entfernungen entlang der Meridiane bleiben ebenso erhalten wie die Entfernungen entlang einer oder zwei Standardparallelen.[2]
	120 (ca.)	Plattkarte oder rektanguläre bzw. rektangulare Projektion = Rektangularprojektion = parallelogrammatische Karte	Marinos von Tyros	Zylindrisch	Flächentreu	Einfachste Geometrie; Entfernungen entlang der Meridiane bleiben erhalten. quadratische Plattkarte: Spezialfall mit dem Äquator als Standardparallele.
	1000 (ca.)	Mittabstandstreue Azimutalprojektion	Abū Rayḥān al-Bīrūnī	Azimutal	Flächentreu	Entfernungen vom Zentrum bleiben erhalten. Wird als Emblem der Vereinten Nationen verwendet, das bis 60° S reicht. Wird vom USGS im Nationalatlas der Vereinigten Staaten von Amerika verwendet.
	1500 (ca.)	Stab-Wernersche Projektion = Werner-Projektion	Johannes Stabius	Pseudokonisch	Flächentreu, Äquidistant	Parallelen sind gleich beabstandete konzentrische Kreisbögen. Die Abstände vom Nordpol sind korrekt, ebenso wie die gekrümmten Abstände entlang der Parallelen und die Abstände entlang des Zentralmeridians.
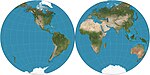	1000 (ca.), 1660	Nicolosi-Kugelprojektion	Abū Rayḥān al-Bīrūnī, neu erfunden 1660 von Giovanni Battista Nicolosi	Pseudokonisch[3]	Kompromiss
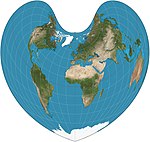	1511	Bonnesche Projektion	Bernardus Sylvanus	Pseudokonisch, herzförmig	Flächentreu	Parallelen sind gleich beabstandete konzentrische Kreisbögen und Standardlinien. Das Aussehen hängt von der Referenzparallele ab. Allgemeiner Fall von Werner-Proj. und sinusförmig.
	1514	Oktanten-Projektion	Leonardo da Vinci	Polyhedral	Kompromiss	Projiziert den Globus auf acht Oktanten (Reuleaux-Dreiecke) ohne Meridiane und ohne Parallelen.
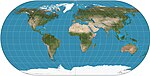	1540	Ortelius ovale Projektion	Battista Agnese	Pseudozylindrisch	Kompromiss	Meridiane sind kreisförmig.[4]
	1569	Mercator-Projektion = Wright	Gerhard Mercator	Zylindrisch	Konform	Linien mit konstanter Peilung (Loxodrome) sind gerade, was die Navigation erleichtert. Die Gebiete blähen sich mit dem Breitengrad auf und werden so extrem, dass die Karte die Pole nicht mehr anzeigen kann.
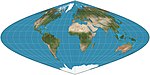	1570 (ca.)	Sinusoidal-Projektion = Sanson-Flamsteed = Mercator flächentreu	(mehrere; der Erste ist unbekannt)	Pseudozylindrisch	Flächentreu, Äquidistant	Meridiane sind Sinuskurven; Parallelen sind in gleichen Abständen angeordnet. Das Seitenverhältnis beträgt 2:1. Die Abstände entlang der Parallelen sind konserviert. Jean Cossin von Dieppe war einer der ersten Kartographen, der das Sinusoidale verwendete, das in einer Weltkarte von 1570 erschien.[5]
	1740	Vertikal Perspektive	Matthäus Seutter *	Azimutal		Ansicht aus einer endlichen Entfernung. Kann nur weniger als eine Hemisphäre darstellen.
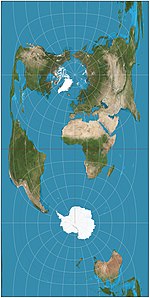	1745	Cassini-Projektion = Cassini–Soldner	César François Cassini de Thury	Zylindrisch	Äquidistant	Quer zur äquidistanten Projektion; die Abstände entlang des Zentralmeridians sind konserviert. Distanzen senkrecht zum Zentralmeridian bleiben erhalten.
	1772	Lamberts Zylinderprojektion	Johann Heinrich Lambert	Zylindrisch	Flächentreu	Standardparallele am Äquator. Seitenverhältnis von π (3,14). Grundprojektion der zylindrischen Gleichflächenfamilie.
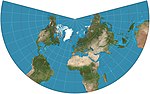	1772	Lamberts winkeltreue Kegelprojektion	Johann Heinrich Lambert	Konisch	Konform	Wird in Luftfahrtkarten verwendet.
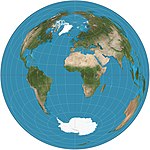	1772	Flächentreue Azimutalprojektion	Johann Heinrich Lambert	Azimutal	Flächentreu	Die geradlinige Entfernung zwischen dem zentralen Punkt auf der Karte und einem beliebigen anderen Punkt ist gleich der geradlinigen 3D-Entfernung durch den Globus zwischen den beiden Punkten.
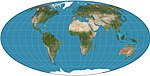	1805	Mollweide-Projektion = elliptisch = Babinet = homolographisch	Carl Brandan Mollweide	Pseudozylindrisch	Flächentreu	Meridiane sind Ellipsen.
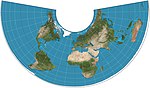	1805	Albers-Kegelprojektion	Heinrich Christian Albers	Konisch	Flächentreu	Zwei Standardparallelen mit geringer Verzerrung zwischen ihnen.
	1820 (ca.)	Amerikanische polykonische Projektion	Ferdinand Rudolph Hassler	Pseudokonisch	Kompromiss	Die Entfernungen entlang der Parallelen bleiben ebenso erhalten wie die Entfernungen entlang des Zentralmeridians.
	1822	Gauss-Krüger-Projektion = Gauss-Konform = (ellipsoidal) transverse Mercator	Carl Friedrich Gauss Johann Heinrich Louis Krüger	Zylindrisch	Konform	Diese transversale, ellipsoide Form des Mercators ist endlich, im Gegensatz zum äquatorialen Mercator. Sie bildet die Grundlage des Universal Transverse Mercator-Koordinatensystems.
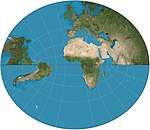	1833	Littrow-Projektion	Joseph Johann von Littrow	Retroazimutal	Konform	Auf der äquatorialen Seite zeigt es eine Halbkugel mit Ausnahme der Pole.
	1850 (ca.)	zentral zylindrische Projektion	(unbekannt)	Zylindrisch	Perspektive	In der Kartographie wegen der starken Polarverzerrung praktisch unbenutzt, aber in der Panoramafotografie beliebt, insbesondere für Architekturszenen.
	1853 (ca.)	rechteckige polykonische Projektion	National Geodetic Survey	Pseudokonisch	Kompromiss	Es kann der Breitengrad gewählt werden, entlang dem der Maßstab korrekt ist. Parallelen treffen rechtwinklig auf Meridiane.
	1855	stereografische Projektion von Gall ähnlich der stereografischen Projektion von Braun	James Gall	Zylindrisch	Kompromiss	Er sollte dem Mercator ähneln und gleichzeitig die Pole zeigen. Standardparallelen bei 45°N/S.
	1855[6]	Gall-Peters-Projektion = Gall’s Orthographic Projection = Peters	James Gall (Arno Peters)	Zylindrisch	Flächentreu	Horizontal komprimierte Version der Lamberts Zylinderprojektion. Standardparallelen bei 45°N/S. Seitenverhältnis von ~1,6. Ähnlich ist die Balthasart-Projektion mit Standardparallelen bei 50°N/S. Karten, die auf der Projektion basieren, werden von der UNESCO gefördert, und sie werden auch von britischen Schulen häufig verwendet.[7]
	1865 (ca.)	Collignon-Projektion	Édouard Collignon	Pseudozylindrisch	Flächentreu	Je nach Konfiguration kann die Projektion die Kugel auch auf einen einzelnen Diamanten oder ein Paar von Quadraten abbilden.
	1879	Quincunx-Kartenprojektion	Charles Sanders Peirce	Andere	Konform	Mosaike. Können kontinuierlich auf einer Ebene gekachelt werden, wobei die Kantenübergänge bis auf vier einzelne Punkte pro Kachel übereinstimmen.
	1887	Guyou-Halbkugel-im-Quadrat-Projektion	Émile Guyou	Andere	Konform	Mosaike
	1889	Aitov-Projektion	Dawid Alexandrowitsch Aitow	Pseudoazimutal	Kompromiss	Dehnung der modifizierten äquatorialen azimutalen Äquidistanzkarte. Die Grenze ist eine 2:1-Ellipse. Weitgehend von Hammer abgelöst.
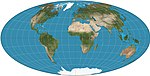	1892	Hammer-Projektion = Hammer-Aitoff Variationen: Briesemeister	Ernst von Hammer	Pseudoazimutal	Flächentreu	Basiert auf der Aitov-Projektion. Geändert gegenüber der azimutalen äquatorialen flächentreuen Äquatorialkarte. Die Grenze ist eine 2:1-Ellipse. Varianten sind schräge Versionen, zentriert auf 45°N.
	1903	Hotine's schiefer Mercator	M. Rosenmund, J. Laborde, Martin Hotine	Zylindrisch	Konform
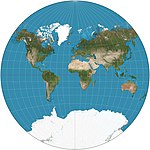	1904	Van-der-Grinten-Projektion	Alphons J. van der Grinten	Andere	Kompromiss	Die Grenze ist ein Kreis. Alle Parallelen und Meridiane sind Kreisbögen. Gewöhnlich nahe 80°N/S beschnitten. Standard-Weltprojektion der NGS in den Jahren 1922–1988.
	1906	Eckert-I- und Eckert-II-Projektion	Max Eckert-Greifendorff	Pseudozylindrisch	Flächentreu
	1906	Eckert-III- und Eckert-IV-Projektion	Max Eckert-Greifendorff	Pseudozylindrisch	Flächentreu	Parallelen sind in Abstand und Maßstab ungleich; äußere Meridiane sind Halbkreise; andere Meridiane sind Halbellipsen.
	1906	Eckert-V- und Eckert-VI-Projektion	Max Eckert-Greifendorff	Pseudozylindrisch	Flächentreu	Parallelen sind in Abstand und Maßstab ungleich; Meridiane sind Halbperioden-Sinuskurven.
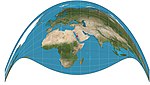	1909	Craig-Retroazimutal-Projektion = Mekka-Projektion	James Ireland Craig	Retroazimutal	Kompromiss	Es handelt sich um eine modifizierte zylindrische Projektion.
	1909	Cahills Schmetterlingskarte	Bernard Joseph Stanislaus Cahill	Polyhedral	Kompromiss	Projiziert den Globus auf ein Oktaeder mit symmetrischen Komponenten und aneinander grenzenden Landmassen, die in verschiedenen Anordnungen dargestellt werden können.
	1910	Hammer-Retroazimutal-Projektion, vordere Hemisphäre	Ernst von Hammer	Retroazimutal
	1910	Hammer-Retroazimutal-Projektion, hintere Hemisphäre	Ernst von Hammer	Retroazimutal
	1910	Behrmann-Projektion	Walter Behrmann	Zylindrisch	Flächentreu	Horizontal komprimierte Version der Lamberts Zylinderprojektion. Hat Standardparallelen bei 30°N/S und ein Seitenverhältnis von 2,36.
	1919	Zwei-Punkt-Äquidistanzprojektion	Hans Maurer	Azimutal	Äquidistant	Zwei „Passpunkte“ können fast willkürlich gewählt werden. Die beiden geradlinigen Entfernungen von einem beliebigen Punkt auf der Karte zu den beiden Passpunkten sind korrekt.
	1921	Winkel-Tripel-Projektion	Oswald Winkel	Pseudoazimutal	Kompromiss	Arithmetisches Mittel der Plattkarte und der Aitoff-Projektion. Standard-Weltprojektion für die NGS seit 1998.
	1922	Roussilhe's schräge stereographische Projektion	Henri Roussilhe
	1923	Goode-Projektion	John Paul Goode	Pseudozylindrisch	Flächentreu	Hybrid aus Sinusoidal- und Mollweide-Projektion. Normalerweise in unterbrochener Form verwendet.
	1925	Adams-Halbkugel im Quadrat	Oscar Sherman Adams	Andere	Konform
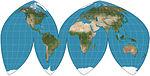	1929	Boggs eumorphe Projektion	Samuel Whittemore Boggs	Pseudozylindrisch	Flächentreu	Die flächentreue Projektion, die sich aus dem Mittelwert der Sinusoidal- und Mollweide y-Koordinaten ergibt und dadurch die x-Koordinate einschränkt.
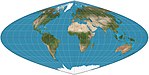	1929	Craster parabolisch =Reinhold Putniņš P4	John Craster	Pseudozylindrisch	Flächentreu	Meridiane sind Parabeln. Standardparallelen bei 36°46′N/S; Parallelen sind in Abstand und Maßstab ungleich; 2:1 Aspekt.
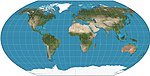	1932	Wagner-VI-Projektion	K. H. Wagner	Pseudozylindrisch	Kompromiss	Äquivalent zu Kawraiski VII vertikal um den Faktor ${\displaystyle {\sqrt {3}}/{2}}$ komprimiert.
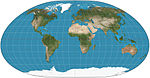	1935, 1966	Loximutale Projektion	Karl Siemon, Waldo Rudolph Tobler	Pseudozylindrisch		Von der angegebenen Mitte aus sind die Linien konstanter Peilung (Loxodrome) gerade und haben die richtige Länge. Im Allgemeinen asymmetrisch um den Äquator.
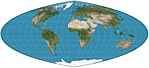	1937, 1944	quartische authalische Projektion	Karl Siemon Oscar Adams	Pseudozylindrisch	Flächentreu	Die Parallelen sind ungleichmäßig in Abstand und Maßstab. Keine Verzerrung entlang des Äquators. Meridiane sind Kurven vierter Ordnung.
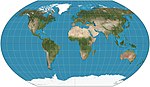	1939	Kawraiski-VII-Projektion	Wladimir Wladimirowitsch Kawraiski	Pseudozylindrisch	Kompromiss	Gleichmäßig verteilte Parallelen. Äquivalent zu Wagner VI horizontal um den Faktor ${\displaystyle {\sqrt {3}}/{2}}$. komprimiert.
	1941	Wagner-VII-Projektion = Hammer-Wagner	K. H. Wagner	Pseudozylindrisch	Flächentreu
	1942	Miller-Zylinderprojektion	Osborn Maitland Miller	Zylindrisch	Kompromiss	Er sollte dem Mercator ähneln und gleichzeitig die Pole zeigen.
	1943	Dymaxion-Projektion = Fuller-Projektion	Richard Buckminster Fuller	Polyhedral	Kompromiss	Weltkarte auf der Oberfläche eines Ikosaeders, die sich entfalten und auf zwei Dimensionen abflachen lässt. Die flache Karte ist stark unterbrochen, um Formen und Größen zu erhalten.
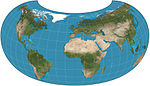	1943	Armadillo-Projektion	Erwin Raisz	Andere	Kompromiss
	1948	Atlantis-Projektion = Mollweide transverse	John Bartholomew	Pseudozylindrisch	Flächentreu	Schräge Version der Mollweide-Projektion
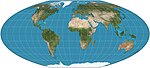	1949	McBryde–Thomas flachpolar-quartic = McBryde-Thomas #4	Felix W. McBryde, Paul Thomas	Pseudozylindrisch	Flächentreu	Standardparallelen bei 33°45′N/S; Parallelen sind in Abstand und Maßstab ungleich; Meridiane sind Kurven vierter Ordnung. Verzerrungsfrei nur dort, wo die Standardparallelen den Zentralmeridian schneiden.
	1951	Hölzel-Projektion = Hölzel-Planisphäre	Verlag Ed. Hölzel	Pseudozylindrisch		Mit ungleich langen parallel gleichabständig verlaufenden Breitengraden, wobei die Pole in Form einer Polarlinie dargestellt werden. Die Längenkreise werden bis zu einer Breite von 80° als Sinuskurven dargestellt, und weiter nördlich ellipsoid zum Pol geführt. Sie ist weder winkeltreu (konform) noch flächentreu, und kein Punkt der Karte ist verzerrungsfrei. Variante der Eckert-V-Projektion; für den Kozenn-Atlas von 1951 erstmals verwendet und in Österreich bis heute verbreitet.
	1953	Bertin-Projektion = Bertin-Rivière = Bertin 1953	Jacques Bertin	Andere	Kompromiss	Projektion, bei der der Kompromiss nicht mehr homogen ist, sondern für eine größere Deformation der Ozeane modifiziert wird, um eine geringere Deformation der Kontinente zu erreichen. Häufig für französische geopolitische Karten verwendet.[8]
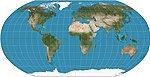	1963	Robinson-Projektion	Arthur H. Robinson	Pseudozylindrisch	Kompromiss	Berechnet durch Interpolation von tabellarischen Werten. Wird von Rand McNally seit seiner Gründung verwendet und von der NGS in den Jahren 1988–1998 eingesetzt.
	1963	Breitenmäßig gleich differentielle polykonische Projektion	China State Bureau of Surveying and Mapping	Pseudokonisch	Kompromiss	Polykonisch: Parallelen sind nicht konzentrische Kreisbögen.
	1965	The Times	John Muir	Pseudozylindrisch	Kompromiss	Standardparallelen 45°N/S. Parallelen auf der Grundlage der Gallischen Stereographie, aber mit gekrümmten Meridianen. Entwickelt für Bartholomew Ltd., The Times Atlas.
	1965	Lee-konforme Welt in einem Tetraeder	L. P. Lee	Polyhedral	Konform	Projiziert den Globus auf einen regelmäßigen Tetraeder. Mosaike.
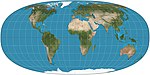	1973	Tobler-Hyperelliptische Projektion	Waldo Rudolph Tobler	Pseudozylindrisch	Flächentreu	Eine Familie von Kartenprojektionen, die als Sonderfälle die Mollweide-Projektion, die Collignon-Projektion und die verschiedenen zylindrischen flächengleichen Projektionen umfasst.
	1973	vierseitiger sphärischer Würfel	F. Kenneth Chan, E. M. O’Neill	Polyhedral	Flächentreu
	1975	Cahill-Keyes-Projektion	Gene Keyes	Polyhedral	Kompromiss	Projiziert den Globus auf ein abgestumpftes Oktaeder mit symmetrischen Komponenten und aneinandergrenzenden Landmassen, die in verschiedenen Anordnungen dargestellt werden können.
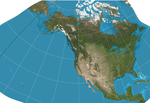	1982	GS50-Projektion	John P. Snyder	Andere	Konform	Speziell entwickelt, um Verzerrungen zu minimieren, wenn alle 50 US-Bundesstaaten angezeigt werden.
	1994	Strebe-1995-Projektion	Daniel „daan“ Strebe	Pseudoazimutal	Flächentreu	Formuliert unter Verwendung anderer flächentreuer Kartenprojektionen als Transformationen.
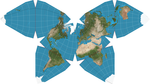	1996	Waterman-Schmetterlingsprojektion	Steve Waterman	Polyhedral	Kompromiss	Projiziert den Globus auf ein abgestumpftes Oktaeder mit symmetrischen Komponenten und aneinander grenzenden Landmassen, die in verschiedenen Anordnungen dargestellt werden können.
	1997	HEALPix	Krzysztof M. Górski	Pseudozylindrisch	Flächentreu	Hybrid aus Collignon + Lamberts Zylinderprojektion.
Link zum Bild	1999	Authagraph-Projektion	Hajime Narukawa	Polyhedral	Kompromiss	Annähernd flächentreu. Mosaike.
	2002	Hobo-Dyer-Projektion	Mick Dyer	Zylindrisch	Flächentreu	Horizontal komprimierte Version der Lamberts Zylinderprojektion. Sehr ähnlich sind Trystan-Edwards- und Smyth-Gleichflächenprojektionen (= Craster-Rechteck) mit Standardparallelen bei etwa 37°N/S. Seitenverhältnis von ~2.0.
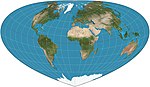	2003	Bottomley-Projektion	Henry Bottomley	Pseudokonisch	Flächentreu	Alternative zur Bonneschen Projektion mit einfacherer Gesamtform Parallelen sind elliptische Bögen. Das Erscheinungsbild hängt von der Referenzparallele ab.
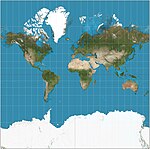	2005	Web Mercator-Projektion	Google	Zylindrisch	Kompromiss	Mercator-Variante, die die Elliptizität der Erde für schnelle Berechnungen ignoriert und die Breitengrade für Präsentationen auf etwa 85,05° reduziert. De-facto-Standard für Web-Mapping-Anwendungen. Sie wird von praktisch allen großen Online-Kartenanbietern verwendet, darunter Google Maps, Mapbox,[9] Bing Maps, OpenStreetMap, Mapquest, Esri, und viele andere.[10]
	2008	Myriahedrale Projektionen	Jarke J. van Wijk	Polyhedral	Flächentreu	Projiziert den Globus auf ein Myriahedron: ein Polyeder mit einer sehr großen Anzahl von Gesichtern.[11][12]
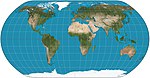	2011	Projektion Natural Earth	Tom Patterson	Pseudozylindrisch	Kompromiss	Berechnet durch Interpolation von tabellarischen Werten.
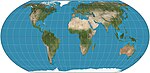	2018	Projektion Equal Earth	Bojan Šavrič, Tom Patterson y Bernhard Jenny	Pseudozylindrisch	Flächentreu	Inspiriert von der Robinson-Projektion, behält aber die relative Größe der Flächen bei.

* Der erste bekannte Popularisierer/Nutzer und nicht unbedingt der Urheber.

Literatur

• John P. Snyder: Map projections – A working manual (= U.S. Geological Survey Professional Paper. Band 1395). U.S. Government Printing Office, Washington, D.C. 1987, Map projections: A working manual, doi:10.3133/pp1395 (usgs.gov [PDF; abgerufen am 4. August 2020]). 
• John P. Snyder, Philip M. Voxland: An Album of Map Projections (= U.S. Geological Survey Professional Paper. Band 1453). U.S. Government Printing Office, Washington, D.C. 1989, doi:10.3133/pp1453 (usgs.gov [PDF; abgerufen am 4. August 2020]). 

Einzelnachweise

1. ↑ Snyder, John P.: Flattening the earth: two thousand years of map projections. University of Chicago Press, 1993, ISBN 0-226-76746-9, S. 1. 
2. ↑ Furuti, Carlos A. Conic Projections: Equidistant Conic Projections
3. ↑ Archivierte Kopie (Memento vom 29. April 2016 im Internet Archive)
4. ↑ Donald Fenna: Cartographic Science: A Compendium of Map Projections, with Derivations. CRC Press, 2006, ISBN 978-0-8493-8169-0, S. 249 (google.com). 
5. ↑ Jean Cossin, Carte cosmographique ou Universelle description du monde avec le vrai traict des vents, 1570.
6. ↑ Gall wird das Verdienst zugeschrieben, die Projektion 1855 auf einem Wissenschaftskongress beschrieben zu haben.
7. ↑ Higgins, Hannah B. The Grid Book. Cambridge, Massachusetts: MIT Press, 2009. ISBN 978-0-262-51240-4 S. 94. „Embroiled in controversy from the start, the map is nonetheless widely used in the British school system and is promoted by the United Nations Educational and Scientific Cultural Organization (UNESCO) because of its ability to communicate visually the actual relative sizes of the various regions of the planet.“
8. ↑ Philippe Rivière: Bertin Projection (1953). In: visionscarto. 1. Oktober 2017, abgerufen am 27. Januar 2020. Vorlage:Cite web/temporär
9. ↑ Our Map Data. MapBox, abgerufen am 20. Juni 2018: „Mapbox supports the popular Web Mercator projection, and currently does not support any other projections for display.“ Vorlage:Cite web/temporär
10. ↑ Sarah E. Battersby, Michael P. Finn, E. Lynn Usery, Kristina H. Yamamoto: Implications of Web Mercator and Its Use in Online Mapping. In: Cartographica. 49. Jahrgang, Nr. 2, 2014, S. 92, doi:10.3138/carto.49.2.2313 (usgs.gov [PDF]). 
11. ↑ Jarke J. van Wijk. "Unfolding the Earth: Myriahedral Projections". [1]
12. ↑ Carlos A. Furuti. "Interrupted Maps: Myriahedral Maps". [2]

Weblinks

• Beschreibung der verschiedenen Kartenprojektionen (Links zu jeder Projektion auf der linken Seite der Seite - auf Englisch)
• Liste der Kartenprojektionen (mit Beispielen) (auf Englisch)
• Visuelle Kartenprojektionsliste